This is the translation of my recent post in Chinese. I was trying to talk in the way that a statistician would use after having stayed along with so many statistics people in the past years.
-------------------------------------------Start----------------------------
Variance is an interesting word. When we use it in statistics, it is defined as the "deviation from the center", which corresponds to the formula 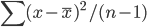 , or in the matrix form
, or in the matrix form 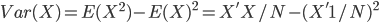 (1 is a column vector with N*1 ones). From its definition it is the second (order) central moment, i.e. sum of the squared distance to the central. It measures how much the distribution deviates from its center -- the larger the sparser; the smaller the denser. This is how it works in the 1-dimension world. Many of you should be familiar with these.
(1 is a column vector with N*1 ones). From its definition it is the second (order) central moment, i.e. sum of the squared distance to the central. It measures how much the distribution deviates from its center -- the larger the sparser; the smaller the denser. This is how it works in the 1-dimension world. Many of you should be familiar with these.
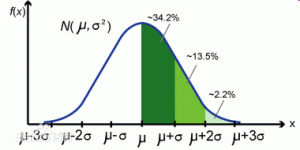
Variance has a close relative called standard deviation, which is essentially the square root of variance, denoted by 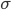 . There is also something called the six-sigma theory-- which comes from the 6-sigma coverage of a normal distribution.
. There is also something called the six-sigma theory-- which comes from the 6-sigma coverage of a normal distribution.
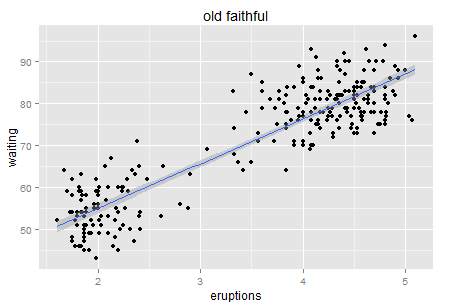
Okay, enough on the single dimension case. Let's look at two dimensions then. Usually we can visualize the two dimension world with a scatter plot. Here is a famous one -- old faithful.
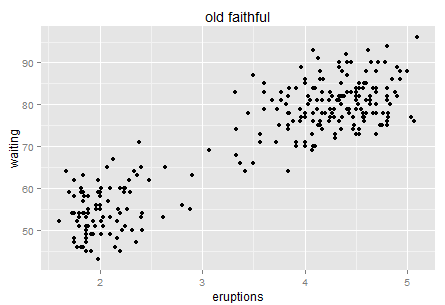 Old faithful is a "cone geyser located in Wyoming, in Yellowstone National Park in the United States (wiki)...It is one of the most predictable geographical features on Earth, erupting almost every 91 minutes." We can see there are about two hundreds points in this plot. It is a very interesting graph that can tell you much about Variance.
Old faithful is a "cone geyser located in Wyoming, in Yellowstone National Park in the United States (wiki)...It is one of the most predictable geographical features on Earth, erupting almost every 91 minutes." We can see there are about two hundreds points in this plot. It is a very interesting graph that can tell you much about Variance.
Here is the intuition. Try to use natural language (rather than statistical or mathematical tones) to describe this chart, for example when you take your 6 year old kid to the Yellowstone and he is waiting for next eruption. What would you tell him if you have this data set? Perhaps "I bet the longer you wait, the longer next eruption lasts. Let's count the time!". Then the kid has a glance on your chart and say "No. It tells us that if we wait for more than one hour (70 minutes) then we will see a longer eruption in the next (4-5 minutes)". Which way is more accurate?
Okay... stop playing with kids. We now consider the scientific way. Frankly, which model will give us a smaller variance after processing?
Well, always Regression first. Such a strong positive relationship, right? ( no causality.... just correlation)
Now we obtain a significantly positive line though R-square from the linear model is only 81% (could it be better fitted?). Let's look at the residuals.
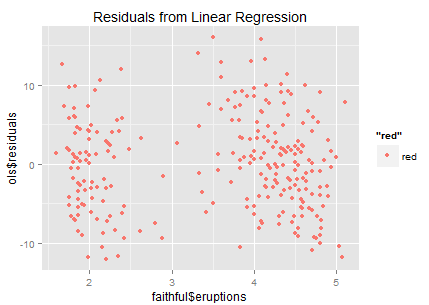 It looks like that the residuals are sparsely distributed...(the ideal residual is white noise which carries no information). In this residual chart we can roughly identify two clusters -- so why don't we try clustering?
It looks like that the residuals are sparsely distributed...(the ideal residual is white noise which carries no information). In this residual chart we can roughly identify two clusters -- so why don't we try clustering?
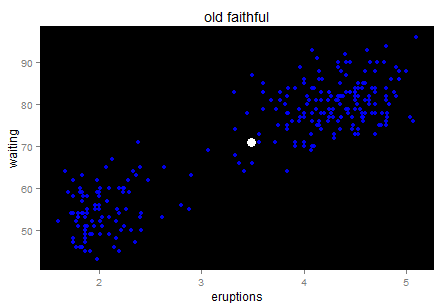
Before running any program, let's have a quick review the foundations of the K-means algorithm. In a 2-D world, we define the center as 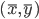 , then the 2-D variance is the sum of squares of each pint going to the center.
, then the 2-D variance is the sum of squares of each pint going to the center.
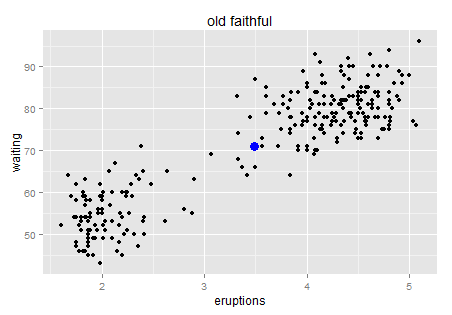 The blue point is the center. No need to worry about the outlier's impact on the mean too much...it looks good for now. Wait... doesn't it feel like the starry sky at night? Just a quick trick and I promise I will go back to the key point.
The blue point is the center. No need to worry about the outlier's impact on the mean too much...it looks good for now. Wait... doesn't it feel like the starry sky at night? Just a quick trick and I promise I will go back to the key point.
For a linear regression model, we look at the sum of squared residuals - the smaller the better fit is. For clustering methods, we can still look at such measurement: sum of squared distance to the center within each cluster. K-means is calculated by numerical iterations and its goal is to minimize such second central moment (refer to its loss function). We can try to cluster these stars to two galaxies here.
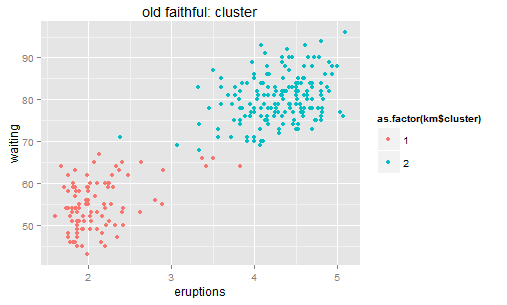 After clustering, we can calculate the residuals similarly - distance to the central (represents each cluster's position). Then the residual point.
After clustering, we can calculate the residuals similarly - distance to the central (represents each cluster's position). Then the residual point.
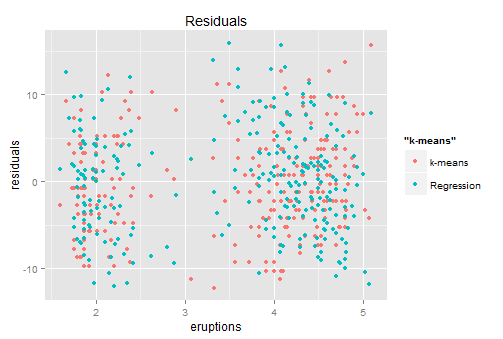 Red ones are from K-means which the blue ones come from the previous regression. Looks similar right?... so back to the conversation with the kid -- both of you are right with about 80% accuracy.
Red ones are from K-means which the blue ones come from the previous regression. Looks similar right?... so back to the conversation with the kid -- both of you are right with about 80% accuracy.
Shall we do the regression again for each cluster?
 Not many improvements. After clustering + regression the R-square increases to 84% (+3 points). This is because within each cluster it is hard to find any linear pattern of the residuals, and the regression line's slope drops from 10 to 6 and 4 respectively, while each sub-regression only delivers an R-square less than 10%... so not much information after clustering. Anyway, it is better than a simple regression for sure. (the reason why we use k-means rather than some simple rules like x>3.5 is that k-means gives the optimized clustering results based on its loss function).
Not many improvements. After clustering + regression the R-square increases to 84% (+3 points). This is because within each cluster it is hard to find any linear pattern of the residuals, and the regression line's slope drops from 10 to 6 and 4 respectively, while each sub-regression only delivers an R-square less than 10%... so not much information after clustering. Anyway, it is better than a simple regression for sure. (the reason why we use k-means rather than some simple rules like x>3.5 is that k-means gives the optimized clustering results based on its loss function).
Here is another question: why do not we cluster to 3 or 5? It's more about overfitting... only 200 points here. If the sample size is big then we can try more clusters.
Fair enough. Of course statisticians won't be satisfied with these findings. The residual chart indicates an important information that the distribution of the residuals is not a standard normal distribution (not white noise). They call it heteroscedasticity. There are many forms of heteroscedasticity. The simplest one is residual increases when x increases. Other cases are in the following figure.
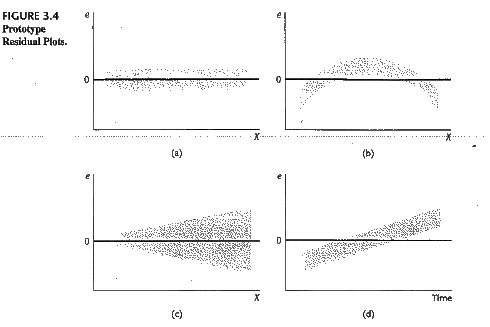 The existence of heteroscedasticity makes our model (which is based on the training data set) less efficient. I'd like to say that statistical modelling is the process that we fight with residuals' distribution -- if we can diagnose any pattern then there is a way to improve the model. The econometricians prefer to name the residuals "rubbish bin" -- however it is also a gold mine in some sense. Data is a limited resource... wasting is luxurious.
The existence of heteroscedasticity makes our model (which is based on the training data set) less efficient. I'd like to say that statistical modelling is the process that we fight with residuals' distribution -- if we can diagnose any pattern then there is a way to improve the model. The econometricians prefer to name the residuals "rubbish bin" -- however it is also a gold mine in some sense. Data is a limited resource... wasting is luxurious.
Some additional notes...